Hi, everyone. Welcome back to another Singapore Maths Academy post. My name is Mohi and today, we’re diving into the world of directed numbers. One of our students recently reached out, needing help with some challenges related to directed numbers. Understandably, directed numbers can be tricky. They’re not something we frequently use in daily life, so it’s easy to get a bit rusty. Don’t worry, though! By the end of this post, you’ll master adding and subtracting negative numbers.
Let’s get started!
Introduction to Directed Numbers
Directed numbers might sound fancy, but they’re essentially numbers with a direction, meaning they can be positive or negative. Understanding directed numbers involves grasping a few foundational rules. Once you’ve got these basics down, tackling more complex problems will be a breeze.
Basic Rules
- Positive Numbers: Simply the numbers we’re most familiar with (e.g., 1, 2, 3).
- Negative Numbers: These are the inverse of their positive counterparts (e.g., -1, -2, -3).
When adding a positive number and its corresponding negative number, they cancel each other out. For instance, (1 + (-1) = 0).
Examples
Let’s break it down with some visual aids and examples.
What is the Negative of 1?
Answer: It’s -1. Simple, right?
Take:
;1 + (-1) = 0;
Summarising the Basics
- (1) is (1)
- (-1) is (-1)
- (1 + (-1) = 0)
These rules are fundamental. They might seem repetitive, but understanding and memorising them is crucial.
Moving to More Complex Numbers
Now that we’ve laid the groundwork, let’s dive deeper.
Adding Various Directed Numbers
Example 1: (3 + (-4))
Step-by-step:
- Start with (3):
- Visualise adding (-4):
Remember, adding a negative number is akin to subtraction:
(3 + (-4) = 3 – 4 = -1).
Here’s another visual representation:

This way, you can see how the negative and positive values cancel each other out, leading to the final answer of (-1).
Further Examples and Visualisation
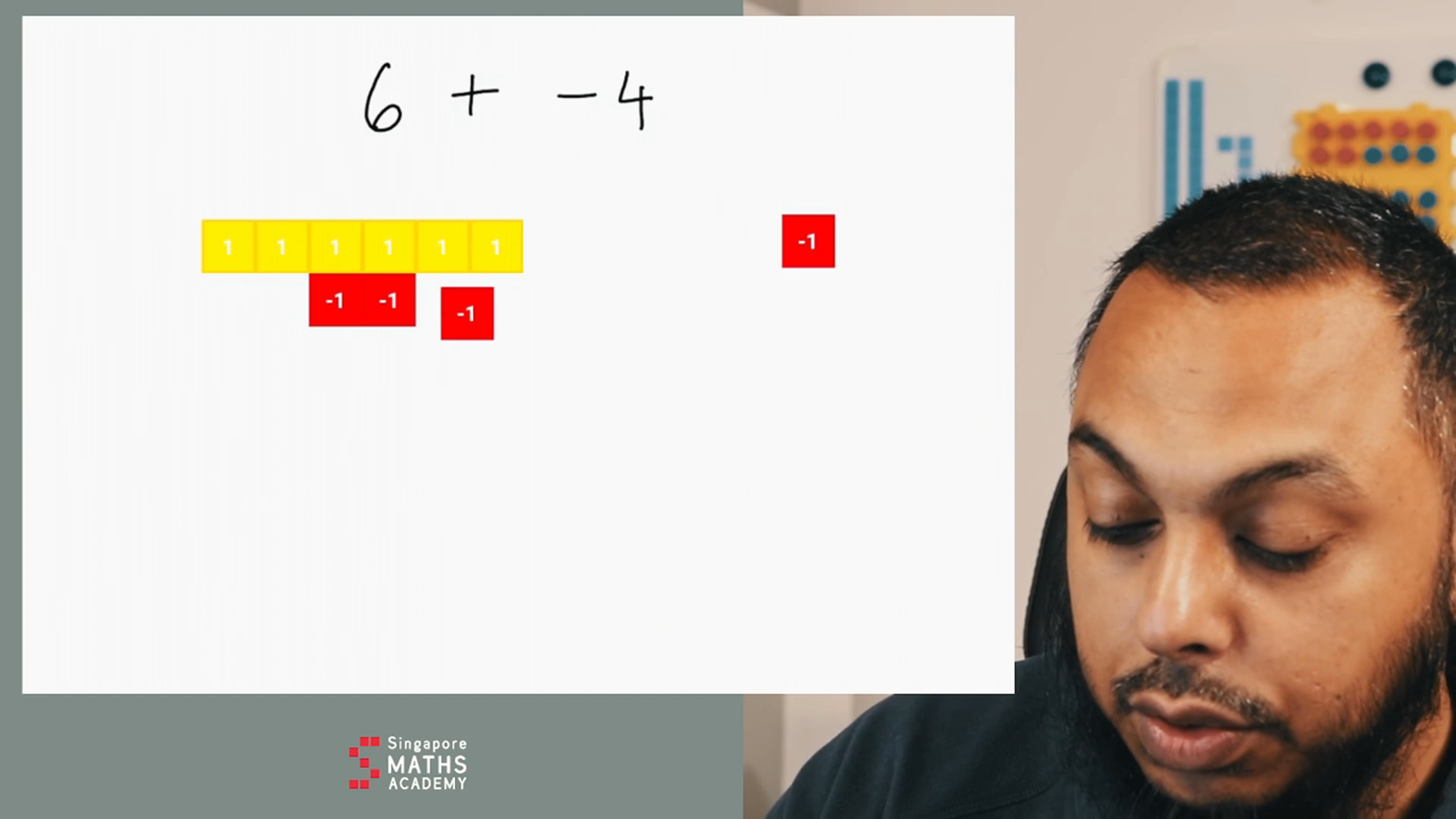
Example 2: (6 + (-4))
- Begin with (6):
- Now add (-4):
With each cancellation, you will be left with (2). Thus, (6 + (-4) = 2).
Adding Negative Numbers
Adding two negative numbers follows a different approach. Let’s explore this.
Example: (-4 + (-5))
Visualising it:
- (-4):
- Now adding (-5):

Together, they form (-9) because:
- (-4 + (-5) = -9)
Understanding through visual aids might help:

Feel free to draw these negatives if needed. Sometimes, writing out the process makes it much clearer!
The “Not Not” Rule
Now, let’s talk about a common sticking point for many students: subtracting negative numbers.
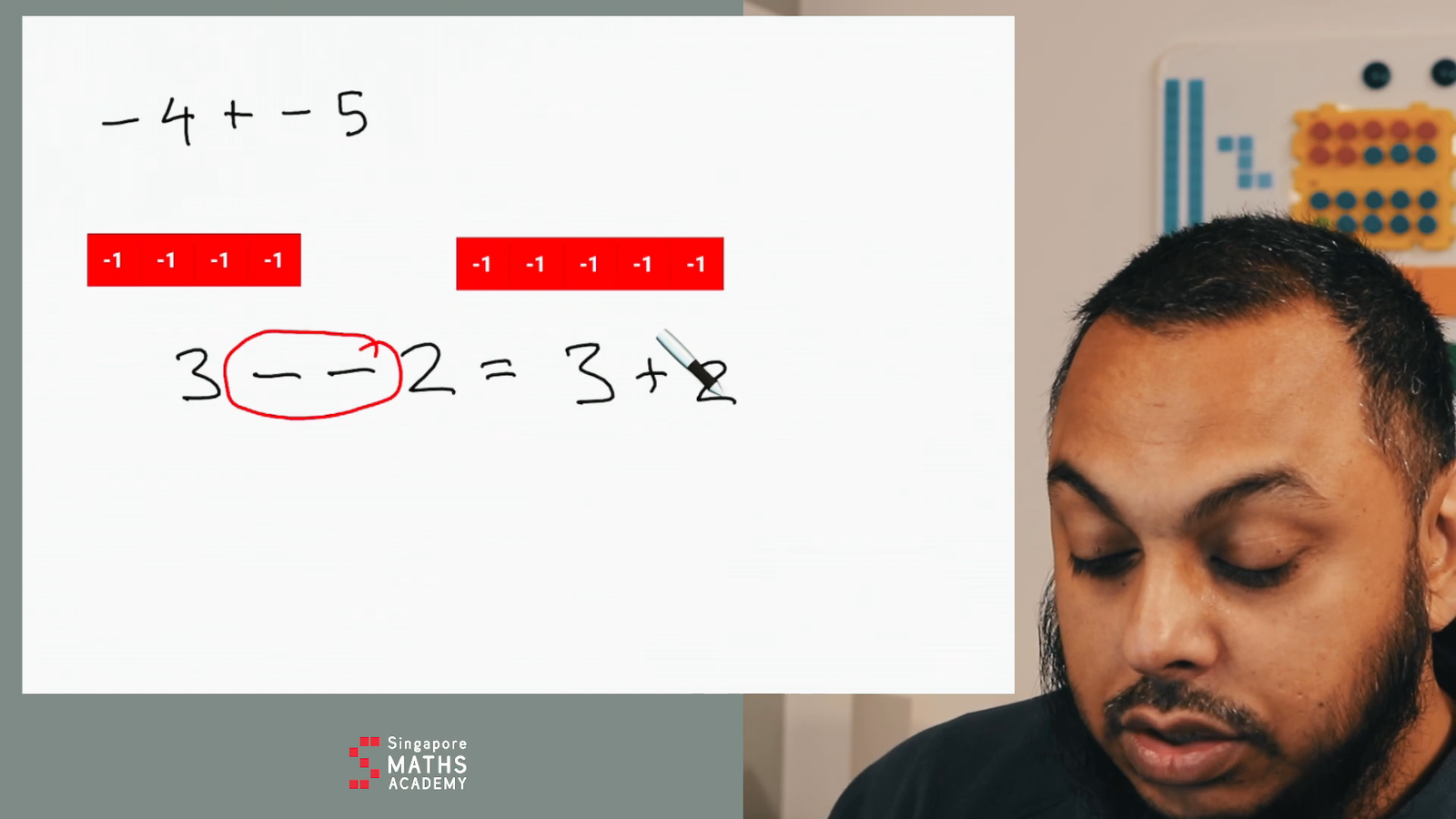
Example: (3 – (-2))
When subtracting a negative, it’s similar to adding the positive counterpart.
- Think of it as the “not not” rule:
- Today is Wednesday. Today is not Wednesday. Today is not not Wednesday (=>) Today is Wednesday.
In our case: (3 – (-2)) transforms to (3 + 2):
3 - (-2) = 3 + 2 = 5A quick visual:
A Few More Examples
Example: (-4 – (-5))
- Begin with (-4):
- Taking away (-5) (i.e., adding (5)):

Thus:
- (-4 – (-5) = -4 + 5 = 1)
By understanding this transformation, directed numbers become much more manageable.
Summary and Practice
To ensure the concept sticks:
- Practice Problems:
- (6 – (-4) = ?)
- (-3 + (-2) = ?)
- Visual Aids:Continue using visuals. They offer immense clarity in understanding the interaction between positive and negative numbers.
Final Thoughts
Grasping directed numbers might seem daunting at first, but with continuous practice and visual aids, it becomes second nature. Remember, whether you’re adding or subtracting, the principles remain consistent.
Stay tuned for our next blog post where we delve into multiplying and dividing negative numbers!
Until next time, happy learning! 😊
Conclusion
Directed numbers are an integral part of mathematics. Although they might appear challenging initially, consistent practice will undoubtedly perfect your skills. If you have any questions or need further clarification, feel free to leave a comment or reach out. We’re here to help!
“Mathematics is not about numbers, equations, computations, or algorithms: it is about understanding.”
– William Paul Thurston