St Olave’s 11+ Maths Questions

Hi, everyone! Welcome to our in-depth exploration of a St Olave’s School eleven-plus maths question. This blog post will walk you through a typical problem you might encounter in the eleven-plus maths pass paper and sample paper. For those finding some questions challenging, this detailed breakdown will be incredibly helpful. Let’s dive right into it!

The Problem

The question we are tackling today reads:

“If each bag of mints weighs 250 grams, how heavy is the cake?”

With a set of balances given, you might not find this question overly challenging at first glance. However, there’s always a way to approach it more efficiently. Let’s explore various techniques to solve this problem.

First Approach: Calculation by Addition

One straightforward method to solve this problem is to simply add up the weights. Here’s how you can do it step-by-step:

1. Identify the Weight of Each Mint Bag: We know from the problem statement that each mint bag weighs 250 grams.
2. Count the Mint Bags: In the given balance, count how many mint bags are there.
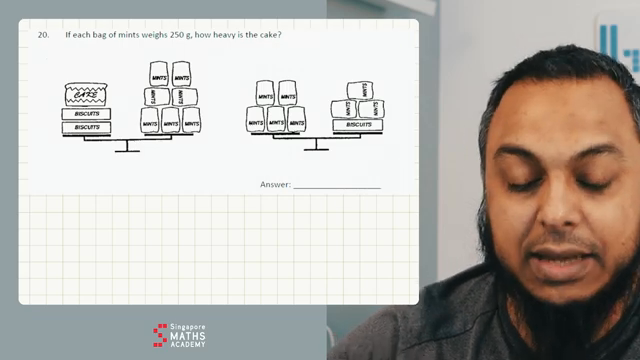
3. Calculate Total Weight: Multiply the total number of mint bags by the weight of one bag.Total Weight = Number of Mint Bags * Weight of One BagFor example, if there are 3 mint bags:Total Weight = 3 * 250 = 750 grams
4. Determine the Cake’s Weight: Next, the cake’s weight can be inferred from the balance.

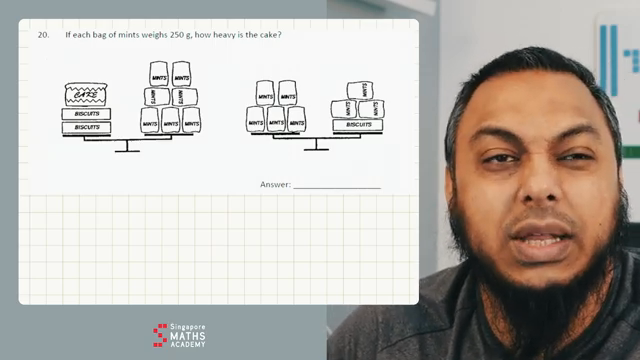
More Efficient Method: Equal Balance Concept

While the first method is direct, a more efficient approach leverages the concept of equal balance. Here’s how:

1. Understanding Balance Equivalence: Notice that if the balances are aligned, the weights on both sides are equal. For instance, if you have three mint bags on one side and three mint bags on the other, the weights are equal because:3 mint bags = 3 mint bags
2. Identify Equal Components: In this case, where two mint bags balance with a biscuit, you can establish that:2 mint bags = 1 biscuit
3. Calculate Individual Weights: Since each mint bag weighs 250 grams:250 grams + 250 grams = 500 gramsHence, each biscuit weighs 500 grams.
4. Compare Equal Weights to Cake: Now, relate the biscuits to the cake:Cake = 3 mint bagsThus, if each mint bag is 250 grams:Cake Weight = 3 * 250 grams = 750 grams

This method emphasizes understanding underlying patterns and relationships, which is a valuable skill in mathematics.

Full Breakdown: Step-by-Step Solution

Let’s break this down further with step-by-step reasoning supported by visuals:

1. Initial Observation: Identify and count all items on the balance. Observe the equal division on both sides.
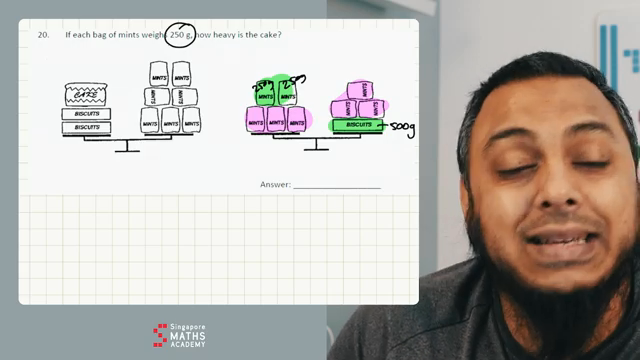
2. Balance Analysis: If each side of the balance has equal quantities, infer that the weights are equal.
3. Component Weight Calculation: Work out the individual weights:2 mint bags = 1 biscuit1 mint bag = 250 grams2 mint bags = 250 grams + 250 grams = 500 grams
4. Applying Weight Equivalence to Cake: Recognize that the cake, balanced against three mint bags, must weigh:Cake = 3 mint bagsCake = 3 * 250 grams = 750 grams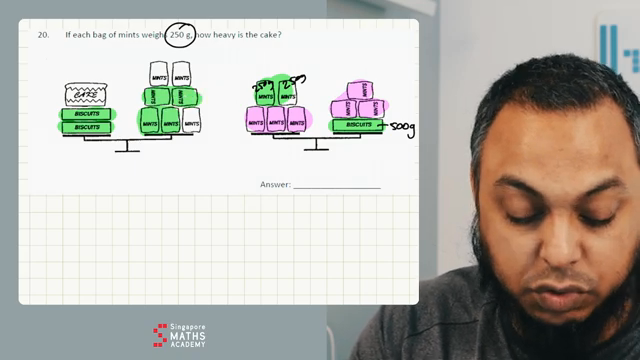By relating these equivalent weights, we achieve quick solutions with minimal calculations.

Application of Efficient Methods

Emphasizing efficiency, especially in timed exams like the eleven-plus, is crucial. By identifying balanced weights and translating this understanding to different components (like mint bags and biscuits), you can save time and reduce potential for error.

Key Takeaways

• Identify Equal Weights: Whenever possible, use balance observations to equate weights before diving into calculations.
• Simplify Calculations: Break down the problem into smaller, manageable pieces. This typically leads to more straightforward solutions.
• Understand Patterns: Recognising patterns and relationships between different items helps simplify complex problems.

Conclusion

In conclusion, mastering problems like the St Olave’s eleven-plus maths question requires both an understanding of basic calculations and the ability to recognise and apply more efficient methods, like balance equivalences. Whether you use direct addition or the balance concept, ensuring you understand the principles underpinning each method is key.

Watch the YouTube video breakdown of this question