In today’s blog post, we’ll dive into an intriguing mathematical concept: multiplying mixed numbers by a whole number. Whether you’re a student brushing up on your skills or an educator looking for a practical way to explain this topic, this guide aims to break it down into simple, understandable steps.
We’ll use an example straight from our hypothetical classroom: Tom used three bottles of syrup to make some desserts, and each bottle contained one and a half litres of syrup. Knowing that one litre of syrup costs five pounds, we will calculate the total cost of the syrup Tom used.
Method 1: Summing up the Syrup
First, let’s understand the total amount of syrup Tom has used.
Step 1: Visualize the Bottles
Tom has three bottles, and each bottle contains one and a half litres. Let’s use some simple representations to keep track.
Bottle 1: | 1.5 litres |Bottle 2: | 1.5 litres |Bottle 3: | 1.5 litres |Step 2: Calculate the Total Volume
To find out the total volume of syrup, we sum up the litres in all three bottles:
- One and a half litres in Bottle 1
- One and a half litres in Bottle 2
- One and a half litres in Bottle 3
1.5 litres + 1.5 litres + 1.5 litres = 4.5 litresSo, Tom has used 4.5 litres of syrup in total.
Step 3: Calculate the Total Cost
We know that each litre of syrup costs five pounds. Therefore, we need to calculate the cost of 4.5 litres of syrup.
First, let’s break down 4.5 litres into manageable pieces:
- 4 litres at 5 pounds each
- 0.5 litres at 5 pounds each
4 litres * 5 pounds = 20 pounds0.5 litres * 5 pounds = 2.5 poundsAdding these together gives:
20 pounds + 2.5 pounds = 22.5 poundsSo, Tom spent 22.5 pounds on syrup.
“I’ve worked out the total amount of syrup I’m using, which is 4.5 litres. Each litre costs five pounds, so the total cost is 22.5 pounds.”
Visual Aid
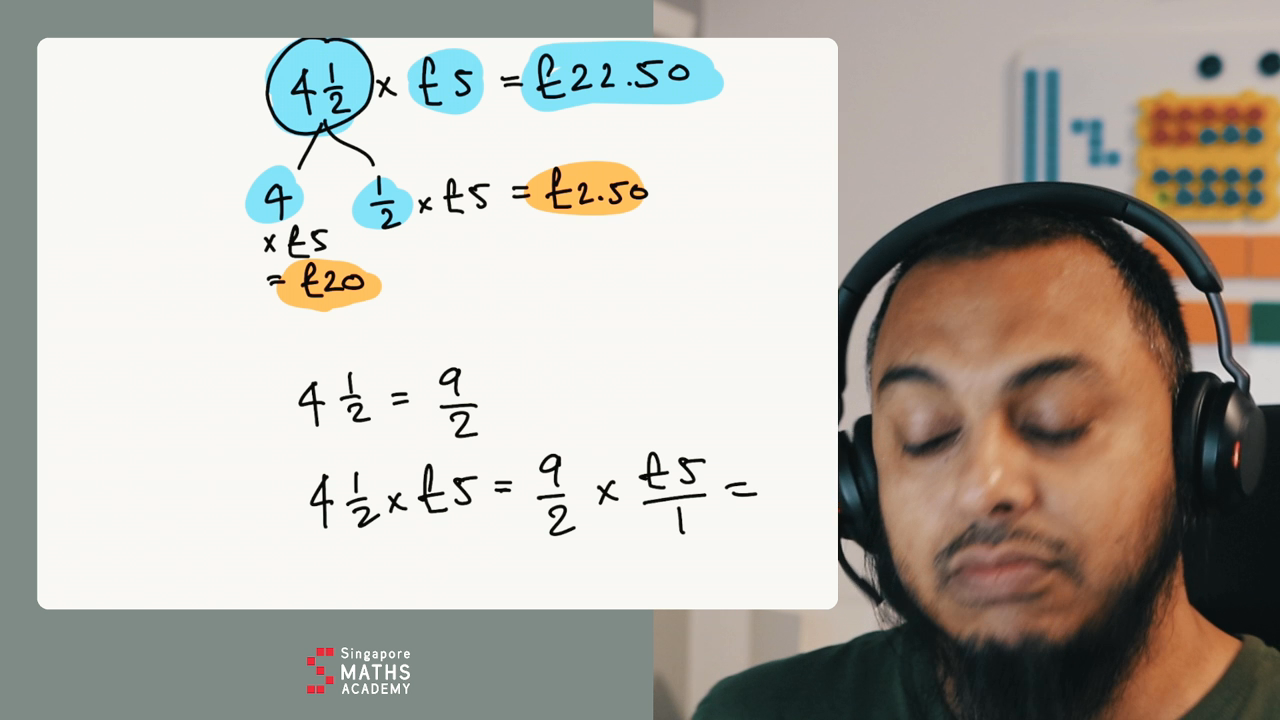
Method 1 (Alternative Approach): Using Fractions
Alternatively, we can use our understanding of fractions to find the cost.
Let’s convert the mixed number 4.5 into an improper fraction.
4.5 = 4 1/2 = 9/2Now, we multiply this fraction by 5 pounds per litre:
(9/2) * 5/1 = 45/2 = 22.5 poundsSo, using fractions, we also find that the total cost is 22.5 pounds.
Method 2: Incremental Calculation
In our second method, we’ll calculate the cost of syrup for each bottle and then sum it up.
Step 1: Calculate the Cost for One Bottle
Each bottle contains 1.5 litres of syrup. We need to calculate how much 1.5 litres of syrup costs.
1.5 litres * 5 pounds per litre = 7.5 poundsSo, each bottle costs 7.5 pounds.
Step 2: Calculate the Total Cost for All Bottles
Now, multiply this cost by the number of bottles:
3 bottles * 7.5 pounds per bottle = 22.5 poundsSo again, we find that Tom spent 22.5 pounds on syrup.
“I calculated how much each bottle of syrup costs, then multiplied by the number of bottles. Each bottle is 7.5 pounds, so three bottles cost 22.5 pounds.”
Summary
We’ve explored two methods for solving our problem of finding the total cost of syrup using mixed numbers and whole numbers. Whether you prefer summing up litres first or calculating incrementally, both approaches lead us to the same answer: 22.5 pounds.
Understanding these methods is crucial as they form the foundation for more complex mathematical concepts. Stay tuned for more math guides, and happy calculating!