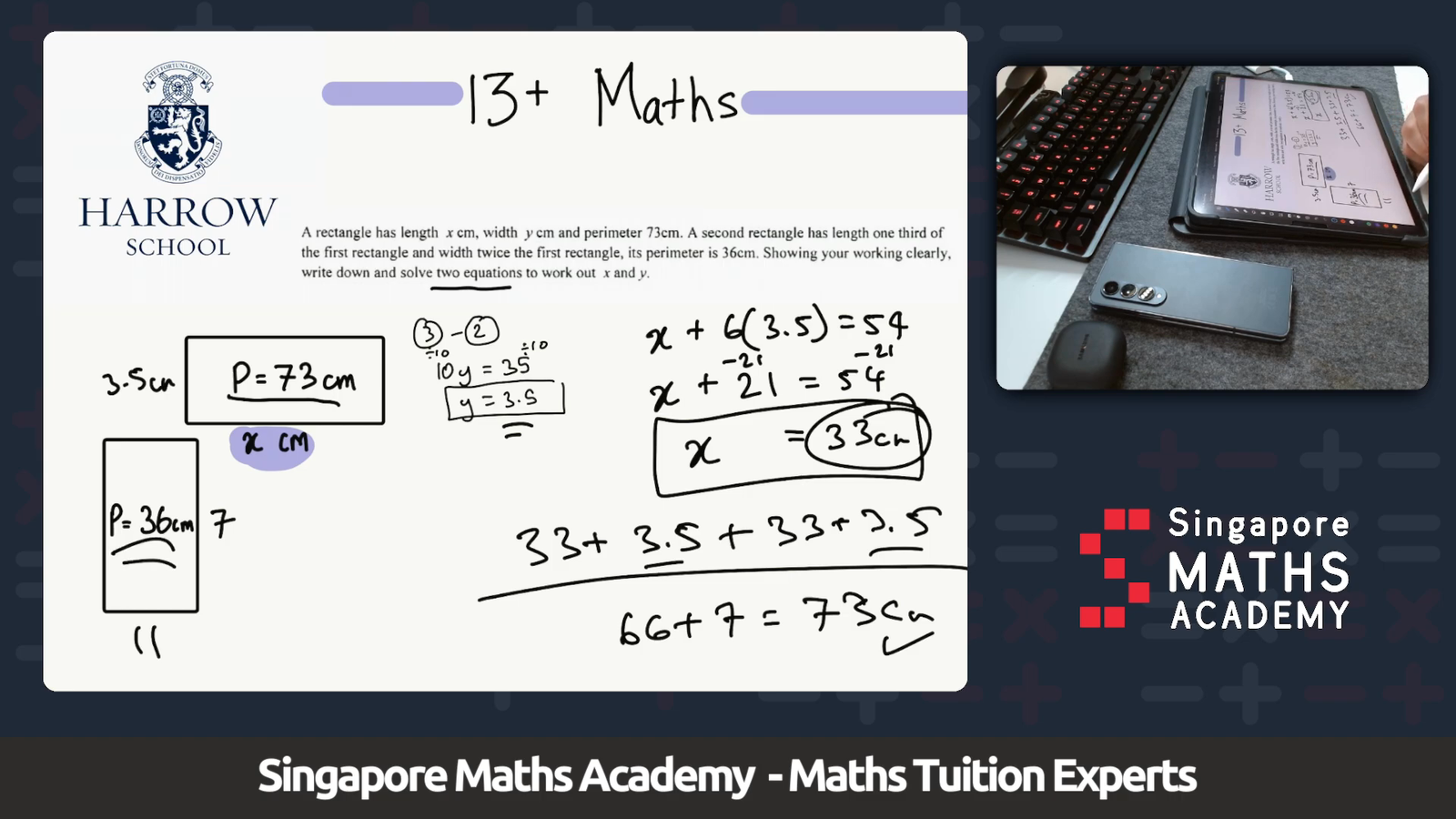
Hey everyone, welcome back to Singapore Maths Academy! Today, we’re tackling a challenging question taken from the Harrow School 13+ entrance exam. Harrow is known for its rigorous admission tests, so this problem is an excellent way to sharpen your mathematical skills. Let’s dive right in and solve this perimeter problem together.
Understanding the Problem
We have two rectangles with given perimeters. The first rectangle has these dimensions:
- Length: ( x ) centimetres
- Width: ( y ) centimetres
- Perimeter: 73 centimetres
Let’s write the first equation for the perimeter of this rectangle:[ 2x + 2y = 73 ]
The second rectangle has these dimensions:
- Length: ( \frac{1}{3}x )
- Width: ( 2y )
- Perimeter: 36 centimetres
Thus, our second equation for the perimeter of the second rectangle is:[ 2 \left( \frac{1}{3}x + 2y \right) = 36 ]
Formulating the Equations
To make it easier to visualise and solve, let’s write down and simplify these equations. Starting with the first rectangle:
First Rectangle
From the perimeter formula of a rectangle (2(x + y) = 73), we divide both sides by 2:[ x + y = 36.5 ]
Second Rectangle
Now, we simplify the second equation:[ 2 \left( \frac{1}{3}x + 2y \right) = 36 ]Divide both sides by 2:[ \frac{1}{3}x + 2y = 18 ]Multiply through by 3 to clear the fraction:[ x + 6y = 54 ]
Now, we have our two equations:
- ( x + y = 36.5 )
- ( x + 6y = 54 )
Solving the Simultaneous Equations
To solve these equations, let’s eliminate one of the variables. We’ll subtract the first equation from the second:
[ (x + 6y) – (x + y) = 54 – 36.5 ]
[ x + 6y – x – y = 17.5 ]
[ 5y = 17.5 ]
Divide by 5:
[ y = 3.5 ]
Now that we’ve found ( y ), we can substitute it back into the first equation to find ( x ):
[ x + 3.5 = 36.5 ]
Subtract 3.5 from both sides:
[ x = 33 ]
So, the dimensions of our first rectangle are:
- ( x = 33 ) cm
- ( y = 3.5 ) cm
Verification
To verify our solution, we substitute ( x ) and ( y ) back into the perimeter formulas to check if they satisfy the given conditions.
First Rectangle
[ 2(33 + 3.5) = 2 \times 36.5 = 73 , \text{cm} ]
Second Rectangle
[ 2 \left( \frac{1}{3} \times 33 + 2 \times 3.5 \right) = 2 \left( 11 + 7 \right) = 36 , \text{cm} ]
Both equations work out, confirming our solutions are correct.
Conclusion
We successfully solved the simultaneous equations to determine the dimensions of the rectangles:
- First Rectangle: Length = 33 cm, Width = 3.5 cm
- Second Rectangle: Length = 11 cm, Width = 7 cm
It’s important to clearly demonstrate your workings in problems like this, especially in entrance exams, as it can help you verify each step and avoid simple errors.
“Success in maths comes from practice, perseverance, and attention to detail.”
Remember to practice similar problems to sharpen your skills further, and good luck with your Harrow School entrance preparation!